We know that angles are said to be complementary if their sum is equal to 90
![]()
.
For example, we have
![]()
![]()
,
![]()
+
![]()
+
![]()
= 180
![]()
We have
![]()
![]()
Therefore,
![]()
and
![]()
are complementary angles.
Lets find out relation between them.
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
From above information we can say that
![]()
![]()
![]()
![]()
![]()
![]()
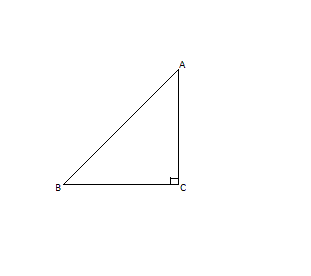
Leave a Reply