CBSE NCERT Solutions Chapter 6 Triangles Exercise 6.3 Question 2
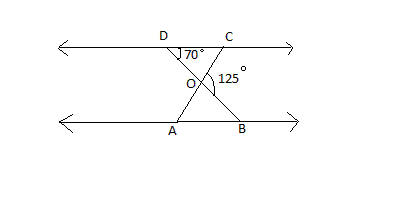
2. In fig 6.35,
![]()
~
![]()
,
![]()
= 125
![]()
and
![]()
= 70
![]()
. Find
![]()
,
![]()
and
![]()
OAB.
Solution:
Given:
![]()
~
![]()
,
![]()
= 125
![]()
and
![]()
= 70
![]()
![]()
+
![]()
= 180
![]()
{Linear Pair}
![]()
= 180 – 125 = 55
![]()
In
![]()
![]()
+
![]()
+
![]()
= 180
![]()
{Sum of angles of Triangle}
![]()
= 180
![]()
It is given that
![]()
~
![]()
.
Therefore, corresponding angles of
![]()
and
![]()
are equal.
By, AAA similarity criterion,
![]()
Therefore,
![]()
Leave a Reply